Calculating credits for green roofs

Recommended pollutant removal efficiencies, in percent, for green roofs.
TSS=total suspended solids; TP=total phosphorus; PP=particulate phosphorus; DP=dissolved phosphorus; TN=total nitrogen

TSS	TP	PP	DP	TN	Metals	Bacteria	Hydrocarbons
85	0	0	0	insufficient data	insufficient data	insufficient data	insufficient data

Download pdf

Page video summary

Warning: Models are often selected to calculate credits. The model selected depends on your objectives. For compliance with the Construction Stormwater permit, the model must be based on the assumption that an instantaneous volume is captured by the BMP.

Green Infrastructure: Green roofs consist of a series of layers that create an environment suitable for plant growth without damaging the underlying roof system. Green roofs create green space for public benefit, energy efficiency, and stormwater retention/ detention.

Credit refers to the quantity of stormwater or pollutant reduction achieved either by an individual best management practice (BMP) or cumulatively with multiple BMPs. Stormwater credits are a tool for local stormwater authorities who are interested in

• providing incentives to site developers to encourage the preservation of natural areas and the reduction of the volume of stormwater runoff being conveyed to a best management practice (BMP);
• complying with permit requirements, including antidegradation (see Construction permit; Municipal (MS4) permit);
• meeting the MIDS performance goal; or
• meeting or complying with water quality objectives, including total maximum daily load (TMDL) wasteload allocations (WLAs).

This page provides a discussion of how green roofs can achieve stormwater credits. Green roofs achieve reductions in stormwater volume compared to conventional roofs. Green roofs can effectively remove or reduce loads of many pollutants that are discharged from conventional roofs, although they may be less effective than other BMPs in removing phosphorus and nitrogen. Green roofs act as vegetated filters and provide temporary storage of rainwater or snowmelt. Water kept in storage may eventually be evapotranspired or "bleed' out of the system to the underlying drainage layer. Water reaching the drainage layer is eventually lost from the green roof system.

Overview

Typical green roof sections. Images from Green Roof Technology

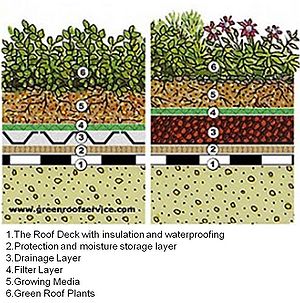
Green roofs consist of a series of layers that create an environment suitable for plant growth without damaging the underlying roof system. Green roofs create green space for public benefit, energy efficiency, and stormwater retention/detention. Green roofs are excellent BMPs in highly urban and ultra-urban environments where it is otherwise difficult to achieve volume and pollutant reductions due to space constraints. Since green roofs release water over a period of time following a precipitation event, they are most effective when discharge from the green roof is to a pervious surface, such as turf or a filter strip. Because green roofs effectively remove sediment, discharge from a green roof can be routed to any BMP (e.g. bioretention, infiltration basin, permeable pavement).

Pollutant removal mechanisms

Green roofs provide filtration of suspended solids and pollutants associated with those solids, although total suspended solid (TSS) concentrations from traditional roofs are generally low. Green roofs provide both volume and rate control, thus decreasing the stormwater volume being delivered to downstream Best Management Practices (BMPs).

Location in the treatment train

Green roofs occur at the beginning of stormwater treatment trains. They may receive discharge from another roof, including conventional roofs, but otherwise do not receive stormwater runoff.

Methodology for calculating credits

This section describes the basic concepts and equations used to calculate credits for volume, Total Suspended Solids (TSS) and Total Phosphorus (TP). Specific methods for calculating credits are discussed later in this article.

Green roofs generate credits for volume by adsorbing water in the planting media. This water may be taken up by plants, evaporate from the media, or be slowly released from the roof. Released water may be routed to a permeable surface, such as a lawn. Although precipitation typically has few pollutants, green roofs can effectively filter many airborne pollutants, including wind blown sediment and pollutants in precipitation, metals and organics. Because the green roof media must support plant growth it typically has a high organic matter content. Green roofs are therefore not effective at removing phosphorus because phosphorus can leach from the organic matter.

Assumptions and approach

In developing the credit calculations, it is assumed the green roof is properly designed, constructed, and maintained in accordance with the Minnesota Stormwater Manual. If any of these assumptions is not valid, the BMP may not qualify for credits or credits should be reduced based on reduced ability of the BMP to achieve volume or pollutant reductions. For guidance on design, construction, and maintenance, see the appropriate article within the green roof section of the Manual.

In the following discussion, the Water Quality Volume (V_WQ) is delivered as an instantaneous volume to the BMP. V_WQ is stored in the media and varies depending on the stormwater management objective(s). For construction stormwater, V_WQ is 1 inch off new impervious surface. For MIDS, V_WQ is 1.1 inches.

Volume credit calculations

Screen shot of MIDS calculator showing inputs used to calculate volume credit for a green roof. Inputs include the media depth and green roof area.

Information: This section does not provide a detailed discussion of green roof hydrology. The article providing an overview of green roofs contains an extensive discussion of green roof hydrology and water retention.

A green roof system acts similar to a bioretention system with an underdrain, although the underlying drainage layer may provide some volume control through storage or by retarding water loss. Volume credits for a green roof system are a function of the dimensions of the system, specifically the depth of the engineered media, the storage and hydraulic characteristics of the media, the area of the green roof, and the amount of run-on from adjacent conventional roofs. The volume credit (V), in cubic feet, is given by

\(V = A_s\ D_p\ (MMWR)\)

where

A_s = the surface area of the green roof(square feet);

D_p = the depth of the media (feet), equal to the area from the bottom of the media (top of underlying drainage layer) to the top of the media; and

MMWR = moisture content at the maximum media density (cubic feet/cubic foot).

The maximum media density (kilograms per cubic meter) is the sample weight (kg) after draining for 120 minutes divided by the initial sample volume (cubic meters). The maximum media density is used to estimate the maximum dead load for green roof assemblies. A value of 0.33 is used for MMWR in the MIDS calculator.

A green roof may be designed to meet a specific performance goal, such as the 1.1 inch Minimal Impact Design Standards (MIDS) goal for new development sites with no restrictions. Specifications for designing green roof systems, including a discussion of how to calculate the reservoir depth, are provided in the section covering design criteria for green roofs. Note that traditional roofs, which are treated as impervious surface, may be routed to a green roof and will therefore affect the achievement of the performance goal. Recommendations about the maximum contributing drainage area from conventional roofs varies. The MIDS calculator limits the size of a conventional roof that drains to a green roof to an area no greater than the area of green roof.

Total suspended solids (TSS) calculations

Since all precipitation falling on a green roof is routed through the media, typical sizing calculations do not apply. Typical roof concentrations of TSS are low, with median concentrations of 10.5 milligrams per liter reported in the International Stormwater Best Management Practices (BMP) Database. The event-based or annual reduction in TSS loading, M_TSS in pounds, is given by

\( M_{TSS} = 2.72\ V\ EMC_{TSS}\ R_{TSS} \)

where

V is the event or annual precipitation, in acre-feet,

EMC_TSS is the event mean concentration in milligrams per liter,

R_TSS is the TSS pollutant removal expressed as a fraction, and

2.72 is a conversion factor.

The recommended removal efficiency is 0.85 (85 percent). Typically, annual precipitation can be used for V when computing the annual TSS reduction. This is because all precipitation passes through the green roof media. It is rare that rainfall intensity is such that water flows across the green roof media.

Example

Assume a 1 inch rain falls on a 1 acre roof. Assume the EMC is 10 milligrams per liter and the removal efficiency is 85 percent (0.85). The TSS removal is equal to 2.72 * 1/12 * 10 * 0.85 = 1.93 pounds.

Total phosphorus (TP) calculations

Since all precipitation falling on a green roof is routed through the media, typical sizing calculations do not apply. Typical roof concentrations of TP are low, with median concentrations of 0.09 milligrams per liter reported in the International Stormwater Best Management Practices (BMP) Database. The event-based or annual reduction in TP loading, M_TSS in pounds, is given by

\( M_{TP} = 2.72\ V\ EMC_{TP}\ R_{TP} \)

where

V is the event or annual precipitation, in acre-feet,

EMC_TP is the event mean concentration in milligrams per liter,

R_TP is the TP pollutant removal expressed as a fraction, and

2.72 is a conversion factor.

Green roofs have been shown to leach phosphorus from the media, although over time they may begin to attenuate phosphorus. The recommended removal efficiency is 0.

Methods for calculating credits

The hydrology of green roofs differs from other BMPs and typical sizing calculations do not apply. There are models and calculators that include green roofs, but generally these models are simplistic and do not simulate green roof hydrology. We recommend using simple models and calculators to calculate credits for green roofs. Examples include the MIDS calculator, Stormwater Management Model (SWMM), EPA SUSTAIN, Green Values National Stormwater Management Calculator, and the Virginia Runoff Reduction Method. Other modeling efforts, specific to green roofs, have been conducted (see, for example, Hilten et al, 2008; Hardin et al, 2012).

For additional information from the literature pertaining to water quality impacts associated with green roofs, we recommend reviewing the technical document prepared for this Manual. This document provides a summary of literature reviewed in development of pollutant removal information for green roofs.

Other pollutants

Although several studies have examined the quality of water discharged from green roofs, few studies have compared results with those from traditional roofs. Traditional roofs are known to leach metals and other materials. Because the green roof media has a high organic matter content, pollutants such as metals and most organic compounds will likely be attenuated in the media. Nicholson et al. provide an overview of water quantity and water quality effects of green roofs compared to traditional roofs, citing limited information that supports retention of several pollutants, particularly metals, by green roofs. For additional reading, see Nicholson et al. (2010) and this abstract by Long et al..

References

• Hardin, M. M. Wanielista, and M. Chopra. 2012. A Mass Balance Model for Designing Green Roof Systems that Incorporate a Cistern for Re-Use. Water. 4:914-931.
• Hilten, R.N., T. M. Lawrence, and E. W. Tollner. 2008. Modeling stormwater runoff from green roofs with HYDRUS-1D. Journal of Hydrology. 358:3–4:288–293
• Nicholson, N., Clark, S., Long, B., Siu, C., Spicher, J., and Steele, K. 2010. Roof Runoff Water Quality—A Comparison of Traditional Roofing Materials. World Environmental and Water Resources Congress 2010: pp. 3349-3355. doi: 10.1061/41114(371)345

Related pages

• Green roofs
  • Overview for green roofs
  • Types of green roofs
  • Design criteria for green roofs
  • Construction specifications for green roofs
  • Assessing the performance of green roofs
  • Operation and maintenance of green roofs
  • Calculating credits for green roofs
  • Cost-benefit considerations for green roofs
  • Plant lists for green roofs
  • Case studies for green roofs
  • Links for green roofs
  • References for green roofs
  • Supporting material for green roofs
  • Green roofs terminology and glossary
  • Green roof fact sheet
  • Requirements, recommendations and information for using green roofs as a BMP in the MIDS calculator
• Calculating credits
  • Calculating credits for bioretention
  • Calculating credits for infiltration basin
  • Calculating credits for infiltration trench
  • Calculating credits for permeable pavement
  • Calculating credits for green roofs
  • Calculating credits for sand filter
  • Calculating credits for stormwater ponds
  • Calculating credits for stormwater wetlands
  • Calculating credits for iron enhanced sand filter
  • Calculating credits for swale
  • Calculating credits for tree trenches and tree boxes
  • Calculating credits for stormwater and rainwater harvest and use/reuse